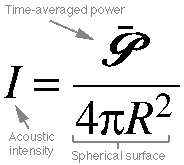
Equation 9.1: The Inverse Square Law. Acoustic energy is radiated from the mouth of a singer as an expanding sphere. As time elapses, this radiated energy gets distributed over a larger and larger area, and so the sound seems softer or weaker to us at a distance. This equation quantifies the decay of that energy. The radius R is the distance of the observer from the sound source. This law gets its name from the fact that intensity is inversely proportional to the radius squared.

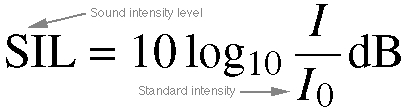
Equation 9.2a. Sound Intensity Level (SIL), or Sound Pressure Level (SPL) as it is usually called, is measured in decibels. The SIL basically tells us how much louder or more powerful a given sound is than a standard (very soft) reference intensity, which is 10^-12 watt/m². This equation defines the sound intensity level, and the next defines SPL:

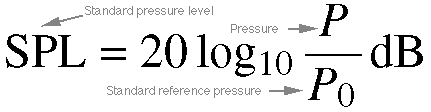
Equation 9.2b. For SPL, a standard reference pressure is used, instead of a standard intensity. P₀ is this reference pressure, which is 20 micropascals (0.00002 Pa). For our purposes, SIL and SPL describe the same level of acoustic energy and can be used interchangeably.

Equation 9.3. If we take Equations 9-1 and 9-2 and substitute in the standard intensity, we get this, assuming a radius of 0.5m. If we assume that a singer is producing 1 watt of acoustic power (the accepted maximum power that human vocalists can produce), then the SPL works out to 115 dB at 0.5m away from the sound source.

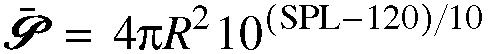
Equation 9.4. If SPL is known and we need to calculate the radiated power, this equation is used.

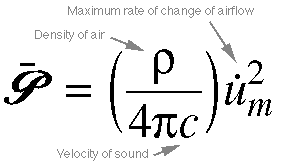
Equation 9.5. This equation describes the power radiating from a piston in a spherical baffle, as shown in Figure 9.2b of the text. This shape is similar to the vocal tract, and so this idealized noisemaker is useful in studying sound production in the voice.

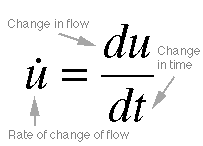
Equation 9.6.

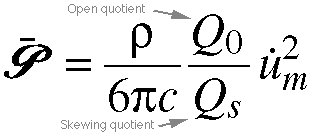
Equation 9.7.

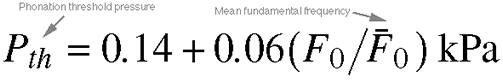
Equation 9.8.

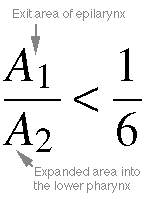
Equation 9.9.