Chapter 6: The Source-Filter
Theory of Vowels

Equation 6.1. Acoustic pressure, p, is
the pressure created by sound waves; acoustic airflow, u,
is the volume of air that moves into the vocal tract
per unit of time. The ratio of these, z, is created.
This is known as the acoustic impedance, which we can
think of as the "effectiveness" of the tube at impeding the
airflow.

Equation 6.2. This equation is derived from
equation 6.1, in the special case where the sound wave
travels in one direction only. In this case, the acoustic
impedance is the ratio of air density, r,
multiplied by sound velocity, c, divided by the
cross-sectional area A.
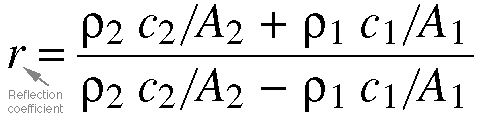
Equation 6.3. The reflection coefficient, r,
is a ratio of the difference between the acoustic impedances
to the sum of the acoustic impedances. This equation
reflects this ratio, with the impedances shown in terms
of equation 6.2.
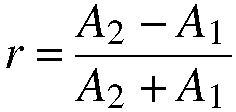
Equation 6.4. Usually, we can assume that the
air density and sound velocity are constant throughout
the airway. This reduces the reflection coefficient to
the ratio of the difference between the areas to the
sum of the areas between adjacent tubes.
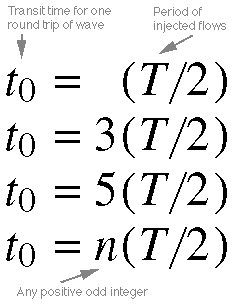
Equation 6.5-8. For any given wave, there is
a value t0 which is the time it takes
a wave to make a complete cycle. This is known as the transit
time.
A standing wave is a waveform that has a finite, discrete
number of nodes. Either endpoint of a standing wave is
fixed at the zero crossing (halfway between the minimum
and maximum amplitudes) or at the minimum or maximum
amplitude.
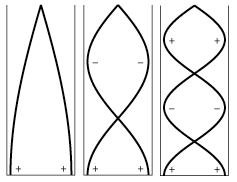
In the case of the open-closed tube shown above, the standing waves follow
the latter case. The first standing waveform has transit time of half the period
of the injected flow waveform. This is displayed in equation 6.5. The second
and third waveform show increasing partial periods. Note that the closed end
amplitude is always a minimum or maximum, while the open end is always at a
zero crossing. The second and third waveforms are shown by equations 6.6 and
6.7, respectively. A noticeable trend beings to appear, which is generalized
in equation 6.8, where n is an odd integer. Graphically, n is
twice the number of "lobes" which are contained within the length of the tube.
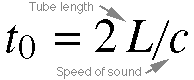
Equation 6.9. Transit time can be expressed
in terms of total distance (two tube lengths) divided
by the speed of sound.
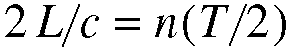
Equation 6.10. For resonance, we can take equation
6.8 and replace the term for transit time with its equivalent
expression defined in equation 6.9.

Equation 6.11. Knowing that the frequency F is
the inverse of the period T, the relation of frequency
to length can be derived from equation 6.10, resulting
in the equation above.
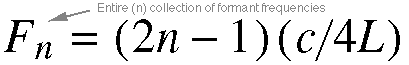
Equation 6.12. A series of frequencies can be
calculated by using different odd integers for n.
These frequencies, which are resonances of the vocal
tract, are known as formants. It is convenient to distinguish
the formants by placing a numerical subscript after the
symbol F. For example, the second formant would
be written as F2. In order to have
a formant n equate to its respective frequency,
it is convenient to write equation 6.11 in terms of the
formant index. The term n from equation 6.11 is
replaced with the expression (2n-1), which results
in equation 6.12, where n is now the formant index.
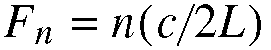
Equation 6.13. Recall earlier than a standing
wave can occupy a tube with two closed ends, two open
ends, or one of each. The last case has been shown in
equation 6.12. However, the formant relation for a tube
with two open or two closed ends is a bit simpler, as
shown above.
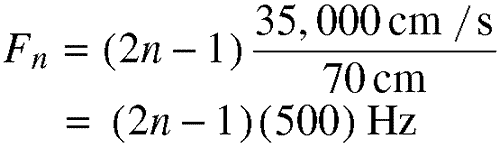
Equation 6.14. To display the practicality of
the formant relation, let us apply some typical values
to the equation. First, assume that the average male
vocal tract length is 17.5 cm. Sound generally travels
through air at a velocity of 35,000 cm/s. Equation 6.14
hence shows a simple equation which can determine the
frequency for an arbitrary formant. The first formant, F1,
is 500 Hz in this case. F2 and F3 follow
at 1,500 Hz and 2,500 Hz, respecitvely.
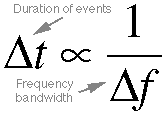
Equation 6.15. As time and frequency are inversely
related, it would follow suit that a duration in time
is inversely proportional to the frequency bandwidth
of the spectrum. Note that as duration of events increase,
the representative bandwidth of frequency decreases.
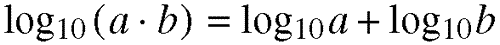
Equation 6.16. This equation demonstrates a
logarithmic property -- the logarithm of a product is
equivalent to the sum of the logarithms of the two factors.
Chapter
5 Equations Equations
Homepage Chapter
7 Equations
|



