Chapter 2: Biomechanics of Laryngeal Tissue

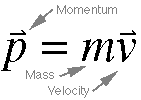
Equation 2.2. Momentum, also a vector quantity, consists of the mass multiplied by the velocity vector. The product is a new vector which measures the potential force (thus the symbol p) when colliding with another object.

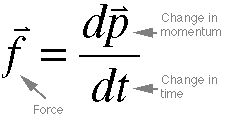
Equation 2.3. Force is defined as the rate of change of momentum over time. Since momentum is made up of mass and velocity, a change in either can change momentum. For our purposes, however, mass usually does not change (the mass of the vocal fold remains constant), and so momentum becomes the product of the constant mass and the rate of change in velocity. This produces:

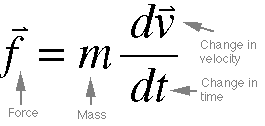
Equation 2.4. This equation has been rewritten to show mass as a static quantity.

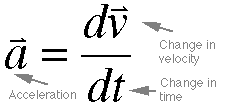
Equation 2.5. Acceleration, also a vector quantity, is the rate of change in velocity over time.

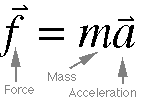
Equation 2.6. If we replace the dv/dt from equation 2.4 with a (equation 2.5 allows us to do this), we arrive at the equation above, which is Newton's Second Law: force equals mass times acceleration.

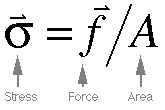
Equation 2.7. Stress, which measures the intensity of a force distributed over a region, is defined as force divided by unit area. This explains why sharp objects can penetrate a hard barrier more easily than dull objects with the same force, because the force is distributed over a smaller area and is thus magnified.

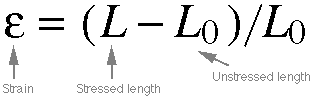
Equation 2.8. Strain is a measure the elongation in a medium resulting from stress being applied to it. It consists of the difference between the original (rest) length and the new (compressed or elongated) length, divided by the orginal length. Strain is someti mes expressed as a percentage (a strain of 0.2 expressed as a 20% elongation, for instance), since it is usually less than 1.0.

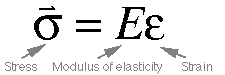
Equation 2.9: Hooke's Law. This law states that stress is proportional to strain. So, any change in one variable will result in a proportional change in the other variable. A doubling of one will result in a doubling of the other, and so forth. E in the equation above is a constant called Young's modulus, or the modulus of elasticity.

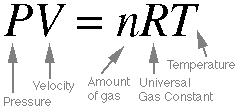
Equation 2.10. This equation describes the behavior of ideal gases. For voice production, the very small pressure differences we create with our voices are the variables to concentrate on. Since the right side of the equation above can be reduced to all constants if we assume a constant temperature, we can use the left side to relate pressure directly to volume, as given in the next equation:

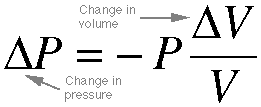
Equation 2.11. The change in volume (delta-V) divided by the unstressed volume V gives us the volumetric strain EPSILON, just as in Equation 2.8 above. This equation can also be written plainly as PV = constant; see Equation 3.1.

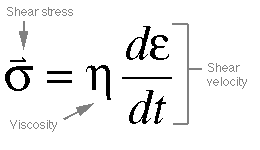
Equation 2.12. This equation governs fluid flow. For a given stress being applied, the larger the viscosity is, the longer it will take for deformation to take place. So, a fluid like molasses would have a rather high viscosity, whereas water or alcohol flow quite freel y and have low viscosities.